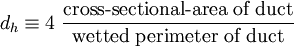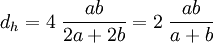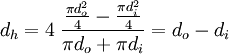Hydraulic diameter
From CFD-Wiki
m |
m |
||
| Line 9: | Line 9: | ||
===Estimating the turbulent length-scale=== | ===Estimating the turbulent length-scale=== | ||
| - | For fully-developed flow in non-circular ducts the [[ | + | For fully-developed flow in non-circular ducts the [[turbulent length-scale]] can be estimated as <math>0.07 \, d_h</math>. This is as usefull estimation for setting [[turbulence boundary conditions]] for inlets that have fully developed flow. |
==Hydraulic diameters for different duct-geometries== | ==Hydraulic diameters for different duct-geometries== | ||
Revision as of 14:03, 24 March 2006
The hydraulic diameter, 
, is commonly used when dealing with non-circular pipes, holes or ducts.
The definition of the hydraulic diamater is:
Contents |
Use of hydraulic diameter
Estimating the turbulent length-scale
For fully-developed flow in non-circular ducts the turbulent length-scale can be estimated as 
. This is as usefull estimation for setting turbulence boundary conditions for inlets that have fully developed flow.
Hydraulic diameters for different duct-geometries
Using the definition above the hydraulic diamater can easily be computed for any type of duct-geometry. Below follows a few examples.
Circular pipe
For a circular pipe or hole the hydraulic diamater is:
Where d is the real diameter of the pipe. Hence, for circular pipes the hydraulic diameter is the same as the real diameter of the pipe.
Rectangular tube
For a rectangular tube or hole with the width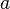 
and the height 
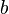the hydraulic diamter is:
Coaxial circular tube
For a coaxial circular tube with an inner diameter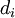 
and an outer diameter 
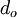the hydraulic diameter is:
