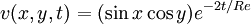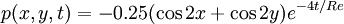Viscous diffusion of multiple vortex system
From CFD-Wiki
(Difference between revisions)
| (2 intermediate revisions not shown) | |||
| Line 1: | Line 1: | ||
| - | The following analytical solution satisfies the incompressible continuity and momentum | + | The following analytical solution satisfies the viscous, incompressible |
| - | equations in dimension-less form in the domain <math>0 \le x, y \le 2\pi</math>. The | + | continuity and momentum equations in dimension-less form in the domain <math>0 |
| - | solution is periodic in both <math>x</math> and <math>y</math> coordinates. | + | \le x, y \le 2\pi</math>. The solution is periodic in both <math>x</math> and |
| + | <math>y</math> coordinates. | ||
:<math> | :<math> | ||
| - | u(x,y,t) = -\cos x \sin y e^{-2t/Re} | + | u(x,y,t) = -(\cos x \sin y) e^{-2t/Re} |
</math> | </math> | ||
:<math> | :<math> | ||
| - | v(x,y,t) = \sin x \cos y e^{-2t/Re} | + | v(x,y,t) = (\sin x \cos y) e^{-2t/Re} |
</math> | </math> | ||
| Line 14: | Line 15: | ||
p(x,y,t) = -0.25( \cos 2x + \cos 2y) e^{-4t/Re} | p(x,y,t) = -0.25( \cos 2x + \cos 2y) e^{-4t/Re} | ||
</math> | </math> | ||
| + | |||
| + | where <math>u,v</math> are the Cartesian velocity components, <math>p</math> | ||
| + | is the pressure and <math>Re</math> is the [[Reynolds number]]. | ||
Latest revision as of 07:40, 12 April 2007
The following analytical solution satisfies the viscous, incompressible
continuity and momentum equations in dimension-less form in the domain 
. The solution is periodic in both 
and
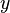coordinates.
where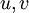 
are the Cartesian velocity components, 
is the pressure and 
is the Reynolds number.
