Hydrodynamic/acoustic splitting
From CFD-Wiki
(→Linearized Perturbed Compressible Equations) |
|||
| (11 intermediate revisions not shown) | |||
| Line 1: | Line 1: | ||
| + | A hydrodynamic/acoustic splitting technique is a good resolving method to the low Mach number aeroacoustics. | ||
In the hydrodynamic/acoustic splitting method, the total flow variables are decomposed into the incompressible variables and the | In the hydrodynamic/acoustic splitting method, the total flow variables are decomposed into the incompressible variables and the | ||
perturbed compressible ones as, | perturbed compressible ones as, | ||
| + | |||
<math>\rho(\vec{x},t)=\rho_0+\rho'(\vec{x},t) </math> | <math>\rho(\vec{x},t)=\rho_0+\rho'(\vec{x},t) </math> | ||
| Line 7: | Line 9: | ||
<math>p(\vec{x},t)=P(\vec{x},t)+p'(\vec{x},t)</math> | <math>p(\vec{x},t)=P(\vec{x},t)+p'(\vec{x},t)</math> | ||
| + | |||
The incompressible variables represent the hydrodynamic flow field, while the acoustic fluctuations and other compressibility | The incompressible variables represent the hydrodynamic flow field, while the acoustic fluctuations and other compressibility | ||
| Line 12: | Line 15: | ||
to calculate the perturbed quantities can be derived by subtracting the incompressible Navier-Stokes equations from the | to calculate the perturbed quantities can be derived by subtracting the incompressible Navier-Stokes equations from the | ||
compressible ones. The PCE recently revised by Seo and Moon are written in a vector form as, | compressible ones. The PCE recently revised by Seo and Moon are written in a vector form as, | ||
| + | |||
<math>\frac{\partial\rho'}{\partial t}+(\vec{u}\cdot\nabla)\rho'+\rho(\nabla\cdot\vec{u'})=0</math> | <math>\frac{\partial\rho'}{\partial t}+(\vec{u}\cdot\nabla)\rho'+\rho(\nabla\cdot\vec{u'})=0</math> | ||
| Line 21: | Line 25: | ||
<math>\frac{{\partial p'}}{{\partial t}}\!+\!(\vec u \cdot \nabla )p'\!+\!\gamma p(\nabla \cdot \vec u') + (\vec | <math>\frac{{\partial p'}}{{\partial t}}\!+\!(\vec u \cdot \nabla )p'\!+\!\gamma p(\nabla \cdot \vec u') + (\vec | ||
u' \cdot \nabla )P = - \frac{{DP}}{{Dt}} + (\gamma - 1)\left( {\Phi - \nabla \cdot \vec q} \right)</math> | u' \cdot \nabla )P = - \frac{{DP}}{{Dt}} + (\gamma - 1)\left( {\Phi - \nabla \cdot \vec q} \right)</math> | ||
| + | |||
| + | |||
| + | where <math>D/Dt = \partial /\partial t + (\vec U \cdot \nabla )</math>, <math>\vec f'_{vis}</math> is the perturbed viscous force | ||
| + | vector, <math>\Phi</math> and <math>\vec q</math> represent thermal viscous dissipation and heat flux vector, respectively. | ||
| + | The PCE are the mixed-scales, non-linear equations, in which terms are involved with coupling effects between the acoustic | ||
| + | fluctuations and the incompressible flow field. Through the coupling effects, a non-radiating vortical component, so-called | ||
| + | 'perturbed vorticity', is generated in the perturbed system. The perturbed vorticity may be regarded as the modification of | ||
| + | hydrodynamic vorticity due to acoustic fluctuations, but it just reside in the perturbed system by this 'one-way' splitting method, | ||
| + | in which backscattering of acoustic fluctuations onto the incompressible flow field is prohibited. | ||
| + | |||
| + | |||
| + | |||
| + | == Linearized Perturbed Compressible Equations == | ||
| + | |||
| + | Although the effects of perturbed vorticity on noise generation is actually negligible at low Mach numbers, it is important to | ||
| + | realize that the perturbed vorticity field can easily become unstable for various reasons. If so, it will get self-excited and | ||
| + | grow unphysically, which then affects the acoustic field and causes inconsistency in the acoustic solutions. Recently, | ||
| + | it is observed that the PCE exhibits grid dependant self-excited errors caused by the instability of perturbed vorticity when | ||
| + | applied to the vortex sound prediction at high Reynolds numbers | ||
| + | |||
| + | In order to resolve the aforementioned matter, the linearized perturbed compressible equations (LPCE) have been proposed by Seo and Moon. The LPCE is a modified version of the original PCE for very low Mach number aeroacoustics. In the LPCE | ||
| + | formulation, the evolution of perturbed vorticity is firmly suppressed by dropping the coupling terms that generate vortical | ||
| + | components in the perturbed system. This is for excluding possible errors caused by its instability, deliberating the fact that | ||
| + | perturbed vorticity is not an important acoustic source for low Mach number flows. A set of LPCE can be written in a vector form as, | ||
| + | |||
| + | |||
| + | <math>\frac{{\partial \rho '}}{{\partial t}} + (\vec U \cdot \nabla )\rho ' + \rho _0 (\nabla \cdot \vec u') = 0</math> | ||
| + | |||
| + | <math>\frac{{\partial \vec u'}}{{\partial t}} + \nabla (\vec u' \cdot \vec U) + \frac{1}{{\rho _0 }}\nabla p' = 0</math> | ||
| + | |||
| + | <math>\frac{{\partial p'}}{{\partial t}} + (\vec U \cdot \nabla )p' + \gamma P(\nabla \cdot \vec u') + (\vec u' | ||
| + | \cdot \nabla )P = - \frac{{DP}}{{Dt}}</math> | ||
| + | |||
| + | |||
| + | The LPCE is linearized to a base incompressible flow field and its formulation is much simpler than the original PCE. | ||
| + | The perturbed vorticity evolution is surely suppressed in the LPCE formulation, since the curl of linearized perturbed momentum | ||
| + | equations, yields | ||
| + | |||
| + | <math> \frac{{\partial \vec \omega'}}{{\partial t}} = 0 </math> | ||
| + | |||
| + | Consequently, the LPCE prevent any further changes (generation, convection, and decaying) of perturbed vorticity in time and a | ||
| + | physical base for large grid spacing near the wall is also established, since they are an invisicd form of equations. The details | ||
| + | about derivation of LPCE and the characteristics of perturbed vorticity can be found in Ref.(Seo and Moon, 2006). | ||
| + | The left hand sides of LPCE represent the effects of acoustic wave propagation and refraction in the unsteady, inhomogeneous flows, | ||
| + | while the right hand side only contains the acoustic source term, which will be projected from the hydrodynamic flow solution. | ||
| + | For very low Mach number flows, it is interesting to note that the total change of hydrodynamic pressure <math>DP/Dt</math> is considered as | ||
| + | the only explicit noise source term. It agrees with the result of Goldstein in his generalized acoustic analogy, | ||
| + | specifically on the linearized Navier-Stokes equations to a 'non-radiating' base flow field. Ewert and Schroder have | ||
| + | also shown that the dominant acoustic source from the flows at the incompressible limit can be represented by the hydrodynamic | ||
| + | pressure. It is also considerable for the turbulent flow noise. The static pressure field of incompressible LES presents the | ||
| + | features of turbulent flow and their noise can be predicted by the LPCE through the time changes of this pressure field. Therefore, | ||
| + | the LPCE could exclude the uncertainty of modeling the source term for flow noise by turbulence. | ||
| + | |||
| + | |||
| + | === Example === | ||
| + | |||
| + | Here are an example of the hydrodynamic/acoustic splitting method. The following figures show Aeolian tone generated by cross flow over a circular cylinder at Re = 180 and Ma = 0.1. The first image is the result of DNS and the next one is the result of Hydrodynamic/acoustic splitting method (incompressible NS/LPCE). The LPCE are computed on the four-times coarser grid. As one can see, the results are almost the same. | ||
| + | |||
| + | [[Image:cyl_180_DNS.jpg|Aeolian tone by cross flow around a circular cylinder at Re=180, Ma=0.1]] | ||
| + | [[Image:cyl_180_LPCE.jpg|Aeolian tone by cross flow around a circular cylinder at Re=180, Ma=0.1]] | ||
| + | |||
| + | == References == | ||
| + | {{reference-paper|author=Seo, J. H. and Moon, Y. J. |year=2005|title=The Perturbed Compressible Equations for Aeroacoustic Noise Prediction at Low Mach Numbers|rest=AIAA Journal, Vol. 43, No. 8, pp. 1716-1724}} | ||
| + | |||
| + | {{reference-paper|author=Seo, J. H. and Moon, Y. J. |year=2006|title=Linearized Perturbed Compressible Equations for Low Mach number Aeroacoustics|rest=Journal of Computational Physics, Vol. 218, pp. 702-719}} | ||
Latest revision as of 22:26, 30 July 2008
A hydrodynamic/acoustic splitting technique is a good resolving method to the low Mach number aeroacoustics. In the hydrodynamic/acoustic splitting method, the total flow variables are decomposed into the incompressible variables and the perturbed compressible ones as,
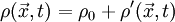
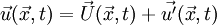

The incompressible variables represent the hydrodynamic flow field, while the acoustic fluctuations and other compressibility
effects are resolved by the perturbed quantities denoted by ('). The original full perturbed compressible equations (PCE)
to calculate the perturbed quantities can be derived by subtracting the incompressible Navier-Stokes equations from the
compressible ones. The PCE recently revised by Seo and Moon are written in a vector form as,
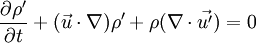


where  ,
, 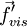 is the perturbed viscous force
vector,
is the perturbed viscous force
vector, 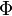 and
and 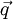 represent thermal viscous dissipation and heat flux vector, respectively.
The PCE are the mixed-scales, non-linear equations, in which terms are involved with coupling effects between the acoustic
fluctuations and the incompressible flow field. Through the coupling effects, a non-radiating vortical component, so-called
'perturbed vorticity', is generated in the perturbed system. The perturbed vorticity may be regarded as the modification of
hydrodynamic vorticity due to acoustic fluctuations, but it just reside in the perturbed system by this 'one-way' splitting method,
in which backscattering of acoustic fluctuations onto the incompressible flow field is prohibited.
represent thermal viscous dissipation and heat flux vector, respectively.
The PCE are the mixed-scales, non-linear equations, in which terms are involved with coupling effects between the acoustic
fluctuations and the incompressible flow field. Through the coupling effects, a non-radiating vortical component, so-called
'perturbed vorticity', is generated in the perturbed system. The perturbed vorticity may be regarded as the modification of
hydrodynamic vorticity due to acoustic fluctuations, but it just reside in the perturbed system by this 'one-way' splitting method,
in which backscattering of acoustic fluctuations onto the incompressible flow field is prohibited.
Linearized Perturbed Compressible Equations
Although the effects of perturbed vorticity on noise generation is actually negligible at low Mach numbers, it is important to realize that the perturbed vorticity field can easily become unstable for various reasons. If so, it will get self-excited and grow unphysically, which then affects the acoustic field and causes inconsistency in the acoustic solutions. Recently, it is observed that the PCE exhibits grid dependant self-excited errors caused by the instability of perturbed vorticity when applied to the vortex sound prediction at high Reynolds numbers
In order to resolve the aforementioned matter, the linearized perturbed compressible equations (LPCE) have been proposed by Seo and Moon. The LPCE is a modified version of the original PCE for very low Mach number aeroacoustics. In the LPCE formulation, the evolution of perturbed vorticity is firmly suppressed by dropping the coupling terms that generate vortical components in the perturbed system. This is for excluding possible errors caused by its instability, deliberating the fact that perturbed vorticity is not an important acoustic source for low Mach number flows. A set of LPCE can be written in a vector form as,
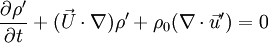
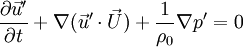
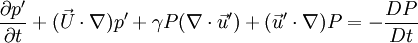
The LPCE is linearized to a base incompressible flow field and its formulation is much simpler than the original PCE.
The perturbed vorticity evolution is surely suppressed in the LPCE formulation, since the curl of linearized perturbed momentum
equations, yields
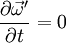
Consequently, the LPCE prevent any further changes (generation, convection, and decaying) of perturbed vorticity in time and a
physical base for large grid spacing near the wall is also established, since they are an invisicd form of equations. The details
about derivation of LPCE and the characteristics of perturbed vorticity can be found in Ref.(Seo and Moon, 2006).
The left hand sides of LPCE represent the effects of acoustic wave propagation and refraction in the unsteady, inhomogeneous flows,
while the right hand side only contains the acoustic source term, which will be projected from the hydrodynamic flow solution.
For very low Mach number flows, it is interesting to note that the total change of hydrodynamic pressure 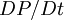 is considered as
the only explicit noise source term. It agrees with the result of Goldstein in his generalized acoustic analogy,
specifically on the linearized Navier-Stokes equations to a 'non-radiating' base flow field. Ewert and Schroder have
also shown that the dominant acoustic source from the flows at the incompressible limit can be represented by the hydrodynamic
pressure. It is also considerable for the turbulent flow noise. The static pressure field of incompressible LES presents the
features of turbulent flow and their noise can be predicted by the LPCE through the time changes of this pressure field. Therefore,
the LPCE could exclude the uncertainty of modeling the source term for flow noise by turbulence.
is considered as
the only explicit noise source term. It agrees with the result of Goldstein in his generalized acoustic analogy,
specifically on the linearized Navier-Stokes equations to a 'non-radiating' base flow field. Ewert and Schroder have
also shown that the dominant acoustic source from the flows at the incompressible limit can be represented by the hydrodynamic
pressure. It is also considerable for the turbulent flow noise. The static pressure field of incompressible LES presents the
features of turbulent flow and their noise can be predicted by the LPCE through the time changes of this pressure field. Therefore,
the LPCE could exclude the uncertainty of modeling the source term for flow noise by turbulence.
Example
Here are an example of the hydrodynamic/acoustic splitting method. The following figures show Aeolian tone generated by cross flow over a circular cylinder at Re = 180 and Ma = 0.1. The first image is the result of DNS and the next one is the result of Hydrodynamic/acoustic splitting method (incompressible NS/LPCE). The LPCE are computed on the four-times coarser grid. As one can see, the results are almost the same.
References
Seo, J. H. and Moon, Y. J. (2005), "The Perturbed Compressible Equations for Aeroacoustic Noise Prediction at Low Mach Numbers", AIAA Journal, Vol. 43, No. 8, pp. 1716-1724.
Seo, J. H. and Moon, Y. J. (2006), "Linearized Perturbed Compressible Equations for Low Mach number Aeroacoustics", Journal of Computational Physics, Vol. 218, pp. 702-719.


