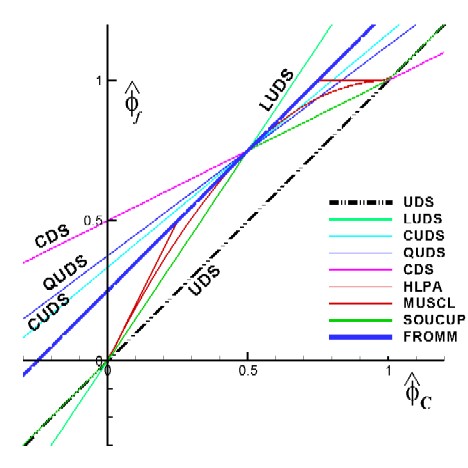
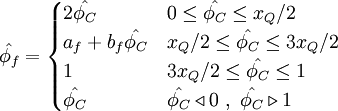Fromm based Schemes - structured grids
From CFD-Wiki
Contents |
Fromm scheme
J.E.Fromm
A method for reducing dispersion in convective difference schemes
J. Comp. Phys., Vol. 3, p.176, (1968)
MUSCL - Monotonic Upwind Scheme for Conservation Laws
Lien F.S. and Leschziner M.A. , Proc. 5th Int. IAHR Symp. on Refind Flow Modelling and Turbulence Measurements, Paris, Sept. 1993
Based on Fromm's scheme
Normalized variables - uniform grids
|
| (2) |
Normalized variables - non-uniform grids
|
| (2) |
where
|
| (2) |
|
| (2) |
van Leer limiter
van Albada
Bounded Fromm
G.D. Van Albada, B.Van Leer, W.W.Roberts
A comparative study of computational methods in cosmic gas dynamics
Astron. Astrophysics, Vol. 108, p.76, 1982
OSPRE
bounded Fromm
Waterson [1995]
N.P.Waterson, H.Deconinck.
A unified approach to the design and application of bounded high-order convection schemes
In C. Taylor and P.Durbetaki, editors, Proc. Ninth Int. Conf. on Numer. Method. Laminar and turbulent Flow, pages 203-214, Pineride Press, Swansea, 1995
Return to Numerical Methods
Return to Approximation Schemes for convective term - structured grids